Number Sense Through the Primary Years
An edited transcript from one of Christina Tondevold’s video clips
Number sense can’t be taught, it’s caught. We cannot directly teach children number sense concepts. Children have to explore, visualize, play with numbers.
When you see a child struggling and you think they are simply not getting it, chances are that these children have no number sense. Children who naturally work fluidly and flexibly with numbers have these number sense concepts.
Early Numeracy Concepts:
Verbal Counting, Object Counting, Cardinality, and Subitizing
Verbal Counting
One of the first things that children start to do when it comes to building their number sense is just learning to count. This is known as verbal counting, the ability to say the counting numbers in the correct order.
When children are first starting out, you will hear them say things such as, one, two, twelve, eleven, thirteen, twenty-four, as though these are just random numbers. They know the difference between numbers and letters but they are not saying it in the correct order.
Object Counting
Knowing the correct order of the numbers does not really tell you that the children understand numbers. They do not necessarily understand that the purpose of being able to count is to count objects.
The next big concept is the need for children to understand that we count for a purpose. When children begin to attach their verbal counts to actual objects and they start touching objects as they’re counting, they are learning that we count for a purpose.
Cardinality
Cardinality is the understanding that once you’ve counted, you then know how many you have. You can answer the question of how many.
Given a picture of 6 items and ask how many items there are to a young child, you may see the child count one, two, three, four, five, six. But then when you ask how many items are there, the child might go right back and count, one, two, three, four, five, six. This child does not understand that six, that last number that is said tells the set.
Verbal Counting in the Upper Primary Years
We begin to count by tens, by hundreds, by twos, by fives. The skip counting we do is helping children build upon their number sense.
Verbal counting does not signify that the children understand it.
If we do a lot of skip counting, that does not really mean the children are attaching meaning to this skip counting. Meaning happens when we start attaching objects and bring in cardinality.
Subitizing

The fourth area of early numeracy is this idea of subitizing. The idea is that at this point children can tell how many there are without having to count.
In this image, you might have some children who have to count to tell you how many apples but if you asked how many fingers is she showing, they know that it’s six instantly and they did not have to count. In that situation, that child is subitizing. Children start seeing things as sets and as groups, and that is a big hinge point into helping your kids move past a counting phase.
Below are some dots. Glance at them for 2 seconds and then turn away and say how many there are.
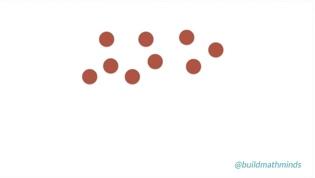
Could you tell how many? Most of us cannot unless you paused it in that moment but in the instant that these dots are flashed, we can’t tell. Even as adults, we cannot subitize more than five unless they are arranged in some kind of nice pattern for us, which is what is what you see below with the same number of dots that are in the picture above.
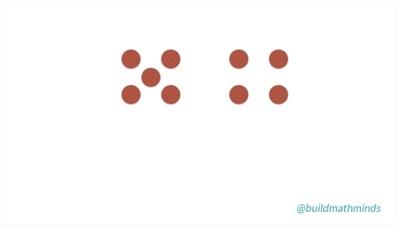
I have organized the dots in a pattern that is familiar.
You really do not see nine, you see a five and a four and then you put those together to help you determine that it was nine. It’s not that you cannot see that nine, you are seeing the smaller pieces within. That’s a big concept of subitizing.
Subitizing in the Upper Primary Years
Here is a picture with more dots. See if you can tell how many there are and think about what mathematical concept this could help develop for our students.

Our instinct is to just tell how many we saw.
What is the mathematical concept that is being representing? Even though I am helping my students build the concept of subitizing, the byproduct is that we are also working on multiplication.
Because multiplication is all about seeing groups. Children need to be able to have these small groupings so that we can use those to help them visualize what multiplication looks like. And it is not just with multiplication, you can do this with multi-digit numbers to really build number sense around those.
We tend to stop using visuals as children start working into multi-digit numbers but children still need the visuals. They need to visualize that 36 is three groups of 10, that it’s 35 and one more, that 36 is four less than 40.
Children need to be able to see those relationships and subitizing helps lay that foundation to allow us to have those conversations.
There is no work on subitizing around fractions but there are visuals. We want children to instantly tell how much is there and as you work into fractions, you can still be doing subitizing around fractions.

We want children to instantly know that that is 1/3.
We want them to instantly know that that’s 1/2.
You lay the foundation with the small amounts and then you work your way into those harder visuals.
Up to now we focused on counting and seeing groups, but the biggest piece of helping children build their number sense is by building relationships.
Number Relationships
Spatial Relationship, One/Two More & Less, Benchmarks, Part-Part-Whole.
Spatial Relationships
Children need a visual to go with a numeral so they can begin to notice relationships. The visual with the dots tells me a lot more about how three relates to four versus if I just have the digits.
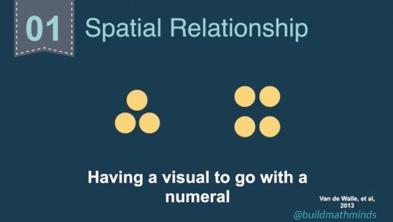

The very first relationship that children start building comes from the visuals and if the only visuals we are providing are numerals, we are doing a disservice to the students.
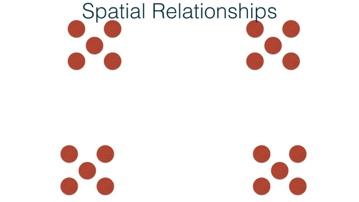
Spatial Relationships in the Upper Primary Years
As we work into multiplication, spatial relationships still apply. Children need that visual. But it’s not just having the visual, it’s how does that visual relates.
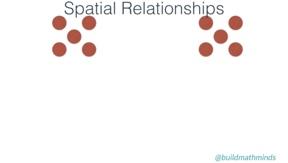
This first visual is 10 but as we work into multiplication, we start to see it as groups of 2 fives. What connections can I help the children start to see here?
One/Two More & Less
Once children have these visuals, then we can start using those to help build the idea of one, two, more and less.
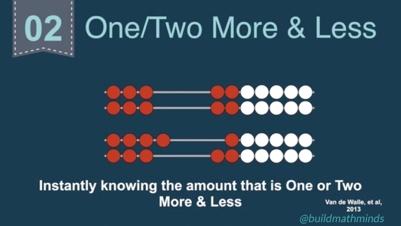
In the picture, I am using a rekenrek. The top rekenrek shows six, the bottom rekenrek shows seven. I can then see the relationship of how six is one less than seven or seven is one more than six.
We too often ask children to fill in worksheets on comparison with the greater than, less than, without providing the child to visualize the digits.

We need those visuals to help children see that relationship. If when they see the digit seven, they can connect it to a visual of a rekenrek or the visual in a 10 frame, or the dot It is helpful to have children be exposed and use many different visuals. When they have those visuals, they can start seeing these relationships. The relationship of one and two, more and less is really, really huge.
One/Two More & Less in the Upper Primary Years

I cannot just tell the children that 35 is one less than 36; I want them to see it.
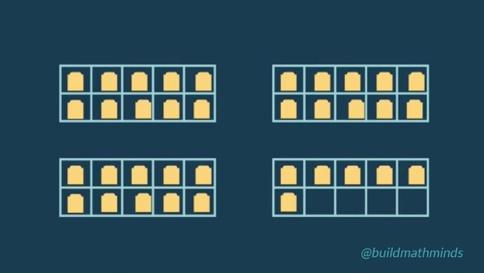
Benchmarks of 5 and 10

The other big relationship in the younger Years is helping children develop the Benchmarks of 5 and 10. Children are often counting out manipulatives individually like the top row up in the picture. But when I count out that top row, it doesn’t tell me anything about how many there are. I can count, I know that there are nine but it does not build anything about the number nine, except it’s one, two, three, four, five, six, seven, eight, nine.
It does not tell me how it relates to five, it doesn’t tell me how it relates to 10 because those benchmark numbers. Letting children put their manipulatives into a 10 frame provides them the opportunity to see how that amount relates to five and how it relates to 10.
It will not be obvious to all of your students, that’s why I say it gives them the opportunity to see that, you have to build that relationship and help them notice some of these things that they might not be noticed on their own.
Benchmarks in the Upper Primary Years
The benchmarks of 1/2 and 1 are huge. When it comes to fractions, benchmark numbers always play a role no matter what Primary Year level.
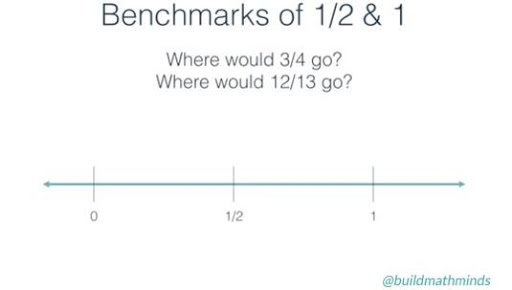
If you are asking children to think about where 3/4 should be placed on the line, I would do not want children to have to chunk that into fours to figure it out. I want them to understand that ¾ is more than a half and less than a whole.
Where would 12/13 on the line? I do not want the children cutting the whole into 13 pieces. I want them to understand that 12/13 is just a little bit under the one on that number line. How about 20/13? I want them to understand that it is more than a whole.

These are big ideas that help with children as they start trying to order fractions from least to greatest. When you see problems like the one above, it is not asking children to find common denominators and to compare them. It is asking children to be able to place the fractions on a number line and use their knowledge of how those fractions relate to the benchmark numbers.
Part-Part-Whole
This is the understanding that if I have seven things, and it is a whole amount, I can break that whole into its parts and I still have the original amount. I can break seven into a one and a six, a two and a five, a three and a four. But again, if I just have the digits there, it’s not building a lot of these relationships for children.

By moving the dots around on a visual, I see I still have the same amount of dots. The children can visualize that the parts come together to make that whole but we can break that whole into different parts and it still gives us the same amount.
Part-Part-Whole in the Upper Primary Years
In our young Years, we start to do activities like decomposing the number five. What are all the ways that you can break apart five? Children will write 0 and 5, 1 and 4, 2 and 3.
Children need to do the same with fractional amounts. How can you decompose 5/8? If it was a one and a four, it’s still a one and a four but it’s 1/8 plus 4/8. If it was a two and a three, it’s 2/8 plus 3/8. How does this help children with addition?
If they are able to decompose five, how would that help them when they are adding nine plus five? If they did that with a fractional amount, could the children decompose 5/8 in a way that would help them add it to 1/2? Can you break it apart? 5/8 is really close to a benchmark number and if you can chunk it in a way that uses those friendly numbers, it makes addition and subtraction so much easier for our children.
When children finally start grasping number sense concepts, it makes operating, whether it’s adding, subtracting, multiplying or dividing, so much easier because children are fluid, flexible, and see connections.
This is just about what makes up number sense. We did not delve into how to do it.
The big thing about how is that you can’t directly teach it. Children need to explore, they need to visualize, they need to play.
Number sense can’t be taught, it’s caught.